[Mechanics] Block on a Ramp
By: Tao Steven Zheng (鄭濤)
【Problem】
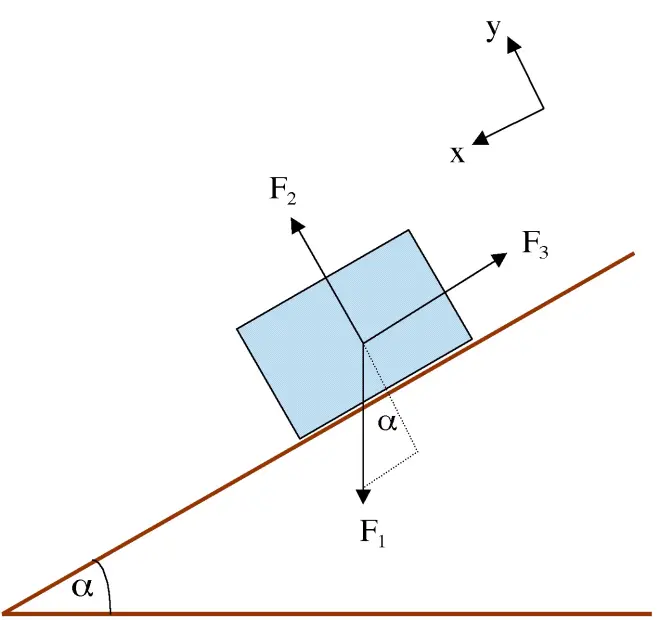
The above free-body diagram illustrates a block of mass? that is stationary on a ramp (inclined plane). The angle of inclination is
, and the coefficient of static friction is
.
Part 1: Identify the forces? in the free-body diagram.
Part 2: Determine the formula for calculating the largest angle in which the block will remain stationary.
Part 3: If the coefficient of static friction is 0.8, what is the maximum angle ?

【Solution】
Part 1
?is the gravitational force
.
is the normal force
.
is the static friction force
.
Part 2
Organize the forces in this problem in two directions: perpendicular to the ramp and parallel to the ramp. Then write the equations of motion in each direction. Note that the gravitational force needs to be resolved into each component; the perpendicular component is
. The parallel component is
.
Perpendicular forces
Since the block is stationary in the perpendicular direction, the acceleration in the perpendicular direction is zero.
Thus,
Parallel forces
Since the block is also stationary in the parallel direction, the acceleration in the parallel direction is zero.
The maximum static friction is calculated using the formula
Thus,
From this equation
Part 3
If the coefficient of static friction is 0.8,

