EEB 122: Principles of Evolution, Ecology and Behavior

Lecture?27.?Interspecific Competition

https://oyc.yale.edu/ecology-and-evolutionary-biology/eeb-122/lecture-27

Last time I was talking about intra-specific competition, and we were looking a bit at the impact of density on population growth. If an individual is encountering increased population density?during its life, as it grows from a zygote up through an adult and reproduces, the effect of increasing density will be to decrease its growth rate. It will be smaller when it matures. It will have fewer babies. Often the babies will be of lower quality, and the mortality rates in the population will be higher. So that's the general impact of intra-specific competition.?

However, animals don't live in a world, and plants don't live in a world, where they only encounter other members of their own species. They're often living in a complex ecological community. And so what we want to do is understand what happens to them in a complex community. So today we're going to talk about?inter-specific competition.

And I'm going to do this by showing you some classical descriptive patterns; then move through to some experiments to demonstrate competition; give you an abstract conceptual framework in which to think about this; and then return to the complex reality of competition, at the end.

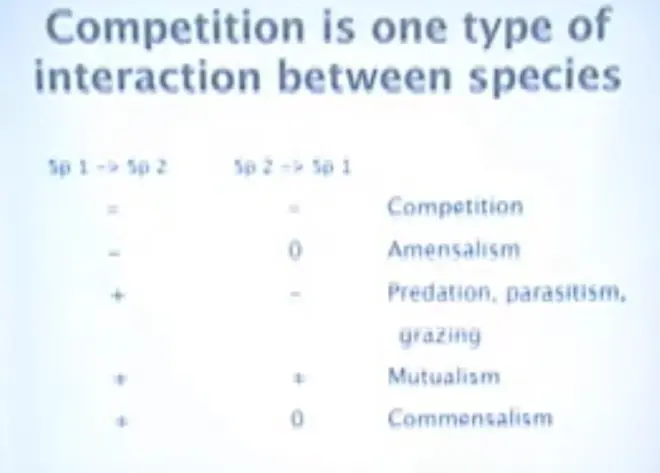
• If species' 1 effect on species 2 is in the first?column, and species' 2 effect on species 1 is in the second?column, and species 1 has a negative effect on species 2, and species 2 has a negative effect on species 1, then we've got competition.

• If species 1 has a positive effect on species 2, and species 2 has a negative effect on species 1, then species 1 is food, and species 2 is eating it, and you've got predation, parasitism or grazing.

• If species 1 has a negative effect on species 2, and species 2 has no effect on species 1, we call that amensalism, and you can contrast that with commensalism, where the relationship is +--0. So here, for example, species 1 might be benefiting--its effect on species 2 is positive. So species 2 is benefiting from its presence, but there's no effect the other way around.

• So basically what's going on here is species 2 might be living in species 1, but not impacting it otherwise. It might be very small or it might not--it might just fit in, in a very comfortable way. So this kind of plus/minus framework is a useful overall way to see what we're concentrating on today, which is competition.?

Here are some of the natural?history?patterns that suggested to ecologists that inter-specific competition is important in Nature. This is one of Jared Diamond's observations,?from?New Guinea. And this is a?thrush. There?are a couple of species of thrush there. There are two species in the same genus. Usually when two species are in the same genus, they're ecologically pretty similar, they tend to eat pretty?similar things.?

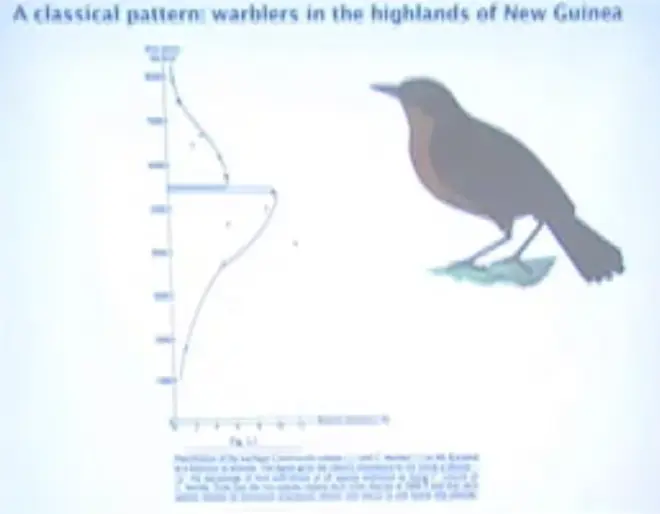
• And the interesting thing about this pattern is that you're going up a mountainside, and this species is getting more and more and more abundant, and then suddenly it disappears. And if you're coming down from the top of the mountain, this species is getting more and more abundant, and then suddenly it disappears, right here at about 5500 feet elevation; just about one mile high. So it looks like the two species are butting into each other and they're excluding each other right at the point where they're each doing really great.

• So that's a suggestive pattern. It doesn't demonstrate that the reason for the border here is inter-specific competition. They each carry a disease that knocks out the other one.?It could be that this one actually can resist a predator that eats its eggs, or something like that, and this one can't resist that predator, and vice-versa.?So it could be apparent competition.?Without an experiment, you don't actually know what is making that sharp line.

• Then Robert MacArthur did a lot of work on warblers for his Ph.D. thesis. And the thing that you want to concentrate on?is the different parts of the spruce tree that the different warblers use.?The warblers of North America have taken the spruce tree and they've divided it up so that they don't run into each other too much, and they're each using a different part. They're guaranteeing that they're going to run into more intra-specific competition.?

Now, those sorts of patterns led Evelyn Hutchinson to suggest this kind of view of why we find so many species on the planet.?

• Over a long period of time evolution will fill the world up with species, and they will get better and better at exploiting the resources they encounter, and competition will limit the number of species you can pack onto the planet.

• At about the time that Hutchinson was thinking about this, various people, including Garrett Hardin, were enunciating what's called the competitive exclusion principle; that two species with very similar resource needs in physiology can't co-exist in the same place, at equilibrium.?That means two species cannot occupy exactly the same nich?and that diversity is determined primarily by competition rather than by predation or by disease or something like that.?

Let's see to what extent this kind of thinking has occasionally been confirmed in Nature.?The pattern that Joe?Connell found--and he confirmed this by doing manipulation experiments in the field--is that the larvae of Balanus, which is big, settle out over a big tidal range. This is mean higher water; this is mean lower spring water; this is mean lower normal tide range. Once a month the tides get this high, and at that point the larvae of Balanus can settle out over the whole tidal range.?

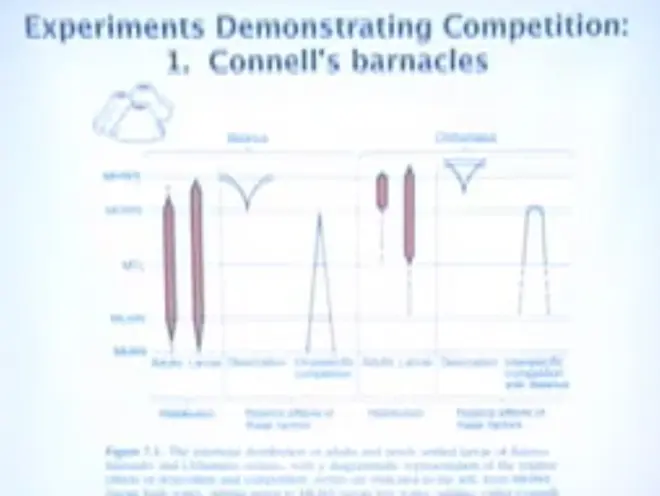
• Balanus is sensitive to drying out, and so as these larvae grow up, many of them die because they're getting desiccated, and so the upper range of Balanus drops. However, it does just fine over the whole tidal range below that. And down here, the problem that Balanus is encountering is that primarily there are other Balanus around crowding them out.

• When two barnacles grow right up next to each other, one of them can actually grow under the other and pry it off; so it'll fall off. So you might think that barnacles look like extremely boring, slow moving rocks, but in fact they have a little bit of direct competitive activity for space.

• Chthamalus is a little guy, and what Chthamalus does basically is it gets a refuge up in the dry part of the upper intertidal. Its larvae can actually settle pretty high up, and it can survive up here, where Balanus cannot. So its problem is primarily getting scrunched by Balanus at lower tidal ranges.

• And by doing cage experiments and removal experiments, where he actually took one type or the other off the rock, and came back--and he actually mapped them out, so he followed each individual--Connell could show that this was what was going on.?

Another famous set of early competition experiments were done by Gause.?And he used three species: Aurelia, Caudatum and Bursaria.?

• What he showed basically is that if you grow them alone, this is their density, that you can measure, per milliliter; and if you grow them together, that Aurelia will exclude Caudatum completely; and that if you compete Caudatum and Bursaria together, they can actually coexist.

• So from these early experiments it did look--by the way, in this circumstance they are both persisting at much lower population densities than when they're alone. So they are depressing each other's densities. They're competing because of that observation. But they manage to coexist, they don't go extinct. So it looked here like there were two possible options. One wins and the other goes extinct, or they co-exist.?

Nelson Hairston did it with salamanders, down in North Carolina, on a huge scale in the appalachian Mountains, and managed to demonstrate that if you pull one salamander out, the density of the other one increases, and they grow faster and they have more babies. So by removing one, you can demonstrate that there is competition going on.?

In all those many experiments, one of the important take-home points is that the results are usually highly asymmetric.?

• That means that the removal of one of the competing species has a much bigger impact on the other; species 1 having a big impact on species 2, and removing species 2 often doesn't have such a big impact on species 1. So asymmetric competition appears to be fairly common.

• And it can get so asymmetric that it may be amensalism rather than competition. So in some cases you get removing one has no impact on the other, whereas you do it the other way around and there's a big impact.

• So there's a continuum of these kinds of cases. And often what's going on is that competition for one resource is reducing the ability of a species to compete for another. So, for example, if plants are competing by shading each other out, then the plant that's getting shaded is having a harder time building roots, which is giving it difficulty getting the water. So you can see there can be a cascade of effects that inter-specific competition might trigger.

• And plants have developed an early warning system to indicate whether they're coming into competition. If they're starting to get shaded by another species, or by another plant, then the ratio of near-red to far-red light, that's coming into their chloroplasts, is getting shifted by being shaded, and they actually produce a hormone, that gives a signal to the plant, oh, I'm getting shaded in that direction, and they will grow a branch out in the other direction; so they'll grow away from shade and toward light, and they actually have an early warning system that's detecting competitions.

Now how to conceptualize all of this? If we take those observations we had, from the last lecture on density dependence,?Verhulst?developed a simple modification of the exponential equation.?

• The key idea there is that as density goes up, the per capita rate of increase is going to decline. So it's going to decline linearly until it reaches 0 at K.

• If density goes up, and when N = K, you have 1 minus 1, which is 0, and that means that the rate of change of population per unit time equals something times 0. So it levels out.?This is increasing, but the rate at which it's increasing is being affected by the density.

• As you get that N closer and closer to K, this part of it's getting closer and closer to 0. So the multiplication rate is dropping and it smoothes right out. In fact, in this simple model, the maximum rate of increase is here, when N is equal to K/2.?

These are the two guys that extended that into multi-species competition: Alfred Lotka and Vito Volterra. They?came up with the same way of conceptualizing these problems.?They've both decided that what they would try to do is basically use the single species framework and just convert the density of the other species into an equivalent number of this species. And that's what these alpha competition coefficients are going to do.?

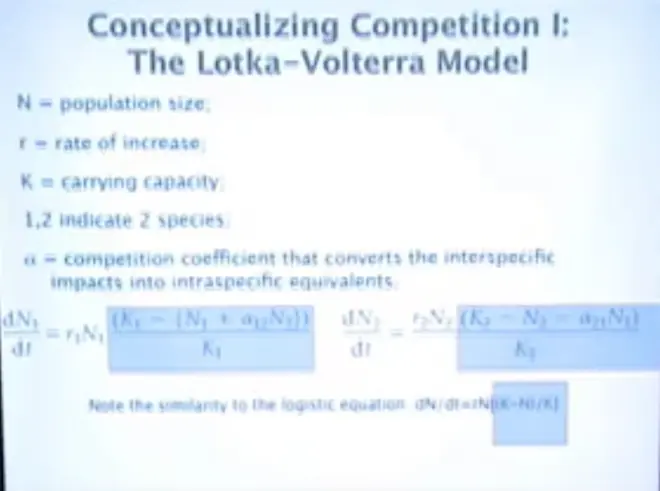
• The rate of change of species 1 is equal to the intrinsic rate of increase of species 1, times the number of species 1, which are present, times a factor that you're using to account for density.

• And the way you account for density is take the carrying capacity of species 1, and you ask, "How far away from that carrying capacity are we?" We have to subtract the number of species 1 which are there.?So we might be a long away from carrying capacity, because we have some of species 1; and we convert species 2 into a number that's equivalent to a number of species 1.

• This is very similar to K - N/K. And you'll see that basically when this number here, N1 + alpha 1, 2 N2 = K1, we have K1 - K1, which is 0/K1. So as this gets larger and larger, the rate of increase is going to smoothly go to 0.?

So they did posit what is probably the simplest way to make that one species situation into a two species situation, and they did it by assuming that you could just convert one species into the other, in terms of its impact on inter-specific competition.?

There are some simple tricks that you can use to get some insight into the dynamic behavior of a system like this, whether you have a numerical solution or not. And so I'm now going to show you that simple trick, and we'll practice it a little bit.?

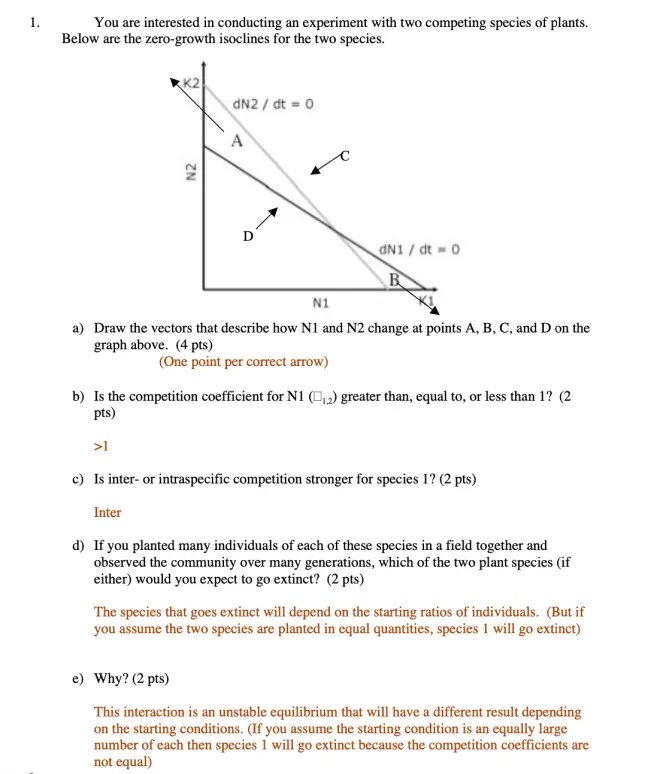
• Here we have plots of N1 versus N2. So what we have done is we've created a phase space.?I am not talking about an axis in which we are plotting the population density of the species against time. I'm talking about axes where we have the population density of one species plotted against the population density of the other species. And time has disappeared, for the moment, from our consideration.

• So this is the zero-growth isocline, and basically what this line here is expressing, at what points is species 1 increasing? And the answer is that if there aren't any species 2 present--so we're down at 0, on the y-axis, no species 2 present--it reverts to the simple logistic model. And species 1 will increase up to the point where it hits its carrying capacity, K. If it goes over that carrying capacity, it will decrease until it hits the carrying capacity. That's the assumption of the model.

• The species 2 isocline intersects the y-axis--not the x-axis but the y-axis--at the carrying capacity of species 2, and if there are no species 1 present, then we're just down to this one axis, and species 2 will increase until it hits its carrying capacity of species 1, and species 2 will decrease if it's over that carrying capacity.?

What the isocline does is it gives you the effects of converting the other species into equivalent competition units for this species. So if you're looking here at the species 1 situation, and you start adding in some species 2, then it will hit its zero-growth point, its carrying capacity, at a lower and lower density of species 1, because there's some species 2 there, that are competing with it. And this line tells you exactly where that will happen. Everywhere along this line species 1 has a zero growth rate.?

Similarly, for species 2. What this line is doing is telling you what the impact of competition, coming in from species 1 is, on species 2. And as you add in species 1, by increasing the numbers out here, it is reducing the density at which species 2 reaches its carrying capacity and its zero growth rate; which is why this line is pointing down here, this line is pointing up here.

And these are the four possible outcomes of competition between two species.?

The first one is that species 1 wins.?

• Species 1 here is always indicated in red, and you can always tell which isocline belongs to which species by looking at where the isocline is intersecting, and how it's labeled. So we know that this isocline belongs to species 1, because the K1 is on the N1 axis. And what you see here is that species 1 is able to grow at higher densities, across the entire range of densities of both species, than is species 2.

• In this intermediate area, between the two lines, species 2 is above its carrying capacity and it's dying out. So basically this is a case where species 1 excludes species 2, completely; it wins. It was that first case we saw in Gause's Paramecia experiments.

The other case is where species 2 wins.?

• The black isocline belongs to species 2. We can tell that by remembering to look for which axis has the K2 on it. K2 is corresponding to N2 here, and it is above the isocline for species 1, over the entire range of possible combinations, of mixtures of both species. And what basically that means is that species 2 can grow at densities where species 1 is declining, and it excludes species 1.?

The more complex, and therefore more interesting cases, are down here. This case is an unstable equilibrium.?

• Above the isoclines, both species are declining. Below the isoclines, both species are increasing. If they happen to be at this point, it's an unstable point, because if they just get a little bit off of it, the system wanders off in this direction and ends up going to be only species 2. And if it wanders a little way off, down here, it goes off in this direction and ends up being species 1.?

And over here you have the interesting case that you have co-existence, and that's where intra-specific competition is stronger than inter-specific competition.

• Above the isoclines they both decrease, and below the isoclines they both increase, and that means that the critical thing to focus on is what's going on in these intermediate triangles here. And if you look at where the carrying capacities are for the individual species, you can see that carrying capacity for 1, in this case, is below the intersection for 2, over here. And that means that above this point, 1 is decreasing and 2 is increasing, and that means that if you plot the change in both of them, you get a vector that points up and to the left.

• And if you look at the relationship of where the intercepts are on the axes, basically you see that this is a situation in which species 1 is being limited by its own density, below the point at which it is being limited by the density of species 2, and species 2 is being limited by its own density, below the point at which it would be limited by the density of species 1. And that tells us that this is a situation in which intra-specific competition is stronger than inter-specific competition.

I don't think the important thing about the Lotka-Volterra equations is that they're an accurate description of reality.?Reality is a lot messier than those equations.?What they are is a good analytical tool that helps you to realize that hey, we're probably dealing with four cases, and there are some nice qualitative ways to simplify the analysis of complex systems of differential equations so that you don't have to do all the numerical calculations.?

There are four outcomes in this case: one wins, two wins, or there's an unstable or a stable equilibrium. And even the very simplest analysis of competition shows that coexistence is possible when intra-specific competition is stronger than inter-specific competition.?

• The resources are discrete and they're scattered across the floor of the Panamanian rainforest, what you have is a concentration of intra-specific competition within each fig. The mating behavior attracts more of your own species to the place where your larvae are going to grow up, and the larvae are competing with each other.

• This process generates a situation in which you get stable coexistence. And there are about 17 species of fruit flies that can live on the same fig tree; the same fruit from the same fig tree, in Panama, and it's very probably because the process I've just described is guaranteeing that intra-specific competition will be stronger than inter-specific competition.?

There's a very interesting result from plant competition experiments that have been done by taking seeds off of individual plants in a meadow like that. What you find is that plants do better in competition with the neighbors than with the average of the larger collection of all species in the meadow. That is something that's really a rather remarkable pattern, because it's almost as though they had been selected to do better against those neighbors. But what process could do that??

• The answer is that there's a seed bank, and that there are seeds of many individuals, from many different species, that are in the soil, all over that meadow, and in the spring when they sprout out, there's selection, and that selection is mediated by competition, and it's acting on seedlings in juvenile stages, and both intra- and inter-specific competition are interacting to determine who survives to be adults. And the ones that we see as adults are the ones that have made it through that competition screen, and if there were twenty or thirty or fifty possible seedlings that were staring up, to yield just one plant, surviving, you can see how that pattern would be generated.

• And so in every generation, out in a meadow, inter-specific competition is mediating the distribution of the plants that we see, and the fact that selection is effective is an indicator that there's a lot of genetic variation within each of those species for competitive ability. This selection couldn't work unless there were genetic variation among individuals in each species. So that each species consists of a whole variety of possible competitive mechanisms. Some of them do well against one neighbor, some of them do well against another; as a result of which you get the maintenance of quite a few different species in the meadow and quite a few different genotypes, within a species, both.?

Okay, so to sum up inter-specific competition. It really does occur. It does help to shape communities in Nature; it's not the only thing shaping communities in Nature, but it's one of the things that shapes them. And it is often asymmetric. So often you have a situation where the bully wins.?

It's frequently been demonstrated, both in field and in lab, and the best way to demonstrate it in the field is to remove one species and see what happens to the other. There is an analytical framework called the Lotka-Volterra model, that helps us to understand and pull together the results of these experiments, and it points us to a key conclusion.?

And the key conclusion is that you'll get coexistence when intra-specific competition is stronger than inter-specific competition. This kind of model, and others, predict that both competitive exclusion and competitive coexistence are possible, depending on the circumstances. And so it was probably a mistake to enunciate the competitive exclusion principle as something that must apply at all times in all places in ecology, because under the particular circumstance of strong intra-specific competition, coexistence is possible; exclusion is not the only logical outcome.