2.5 Measurement

It is assumed that a Lotus Type 79 contains 4 parts to generate downforce: the front wing, rear wing, body, and chassis. Therefore, each of them is put on the mass scale to determine the downforce they produce. The configuration is similar to as follows:

2.5.1 Front Wing

The mass of the front wing is measured first. Its mass is too small, so a heavier mass will be put beside it.

The mass of the heavy mass is 39g, so the mass of the wing is 2g.

In the wind tunnel, the heavier object will be placed beside the front wing to minimize the effect on it.

When testing wings, it is important to take angle of attack accountable. Since the edges of the front wing should be parallel to the ground on the actual car, it can be directly put on the mass scale without any further support since the angle of attack would be the same as that on the real car.

Before testing, it is made sure that the mass scale does not show a nonzero weight when only itself is put in the tunnel.

?

The weight shown on the balance, W, is represented by:

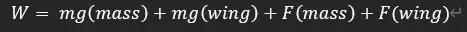
Where mg(mass) is weight of the heavy mass, F(wing) is that of the wing, F(mass) is downforce exerted on the mass, and F(wing) is downforce on the front wing.

mg(mass) and mg(wing) are already obtained as their weight is known, F(mass) plus mg(mass) is as follows, where the mass only is put on the scale and blown:

After putting the wing, the weight should be sum of weight and downforce of both objects:

Therefore, the downforce on the front wing, F(wing), is:

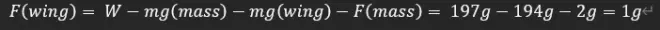
2.5.2 Rear Wing

The rear wing’s bottom is not flat, but its top end is flat and is parallel to the ground on the actual car. Therefore, it is better to place the rear wing upside down and measure the lift force.

The mass of the rear wing is 43g-39g = 4g.

The reading of weight as the balance is in the tunnel before and after putting the rear wing is:

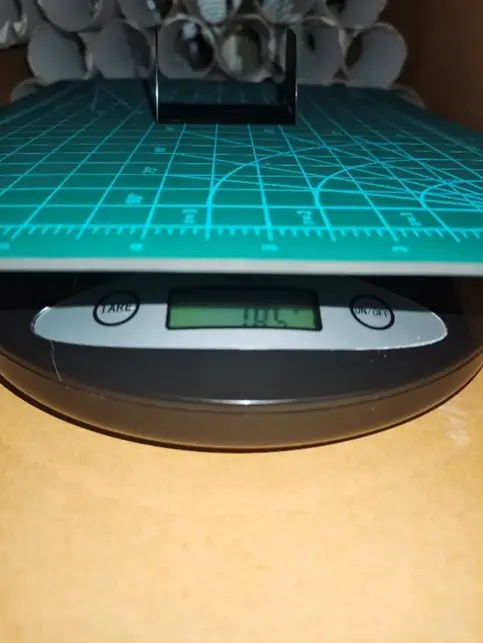
The weight on the right side is represented by:

So, downforce of the wing, F(wing), is:

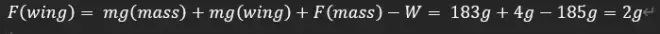
2.5.3 Body

Since the body’s mass is large enough, it does not need the mass anymore.

The mass of the body is as follows:

The reading of weight as the balance is in the tunnel before and after the car is put is:

The weight on the right side is represented by:

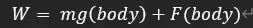
Where mg(body) is weight of body and F(body) is downforce on the body.

It is worth noticing that the weight of the body in the tunnel is less than that without the air blowing. This means that F(body) is negative, indicating that the force on the body is directed vertically upward. Rather than downforce, the body produces lift force. This is actually explainable using the Bernoulli equation and Kutta condition.

Kutta condition can be concluded as that, for a wing to produce lift force, the flow must join at the end of the wing. So, when two air particles hit the wing at the same moment, but as one circles above the wing and the other below, they will meet at the end of the wing. The body is approximated into a wing in this occasion, and if a flow will join at its end, then the particles above the body will have to travel faster than those below since the distance above the body is larger.

Then, the Bernoulli’s equation could be applied. It is given as follows:

And in aerodynamics, as change in height is relatively small, it is often approximated into:

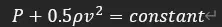
Assume there are two particle hitting the body at the same time, but one goes above the body and the other goes below. Bernoulli’s equation applies for both of them. Therefore, the bigger v, the speed of the air particle, is, the smaller the P, pressure the particle experiences, would be. Therefore, as particles above the body travel with a larger speed, they experience less pressure than the particles below the body. This pressure differences results in a vertical upward force, creating lift force.

In this manner, the body actually resembles a lot to an actual airfoil used on planes---airfoils’ upper end has larger distance so that the particles above it travels faster, creating lift force. Both the airfoils and the body in this case produce a lift force using pressure difference.

This explains why most old road cars without special aerodynamic design experience slight lift force at high speeds---their body also has a larger distance, resulting in a pressure difference that cause an upward force.

This explains why F1 cars even prior to 2022, the season when ground-effect “returns,” contains Venturi tubes on their chassis---they function as tubes even though they are just wings, as shown below, and as the tubes become narrower, they speed up the flow below the racecar.

As the air below gets faster than that above, the pressure below becomes smaller, generating downforce. In this way, a Venturi tube turns a car from an upright airfoil producing lift force into an inverted airfoil producing force vertically downward.

?

So, downforce of the body, F(body), is:

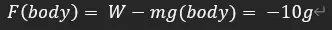
This means the body of the model car generates 10g of lift force.