EEB 122: Principles of Evolution, Ecology and Behavior

Lecture 29. Island Biogeography and Invasive Species

https://oyc.yale.edu/ecology-and-evolutionary-biology/eeb-122/lecture-29

What we're looking at now is the impact of space on communities and on the distribution of plants and animals around the planet. So I'm going to talk today about island biogeography and metapopulations. And I want you to start thinking of the world as fragmented,?as spatially heterogeneous. There are islands, there are mountains, there are lakes and oases.?

And a lot of the world is becoming fragmented by humans. So one of the big ways that humans are having impact on the planet, and changing life on the planet, is by fragmenting the landscape. One?kind of natural fragmentation, of course, is archipelagos, islands. Now we're getting towards island biogeography. The bigger the island the more the species.?

Let's suppose that there?isn't any evolution going on, and that?all of the species we're considering?already exist on?some?big continental landmass, and that they're getting onto islands in a process of?immigration, and they're dying out on islands in a process of?extinction. And this is the number of species that are present on the island.

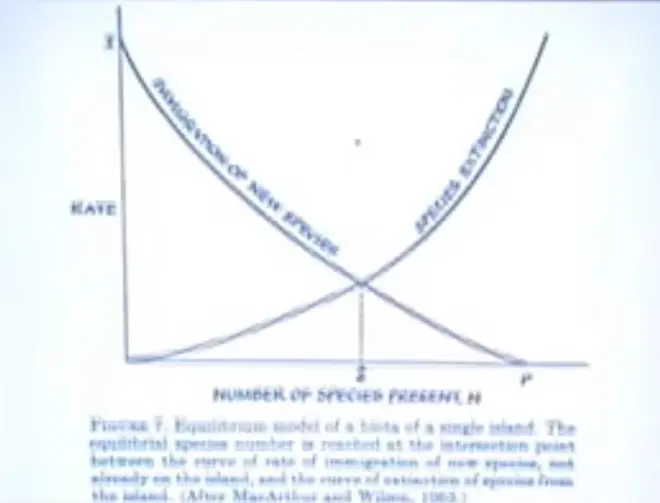
• In such a situation the number of species on the island will come to an equilibrium--there'll be species that are coming off of the continents, that are flying, drifting, getting blown?onto the islands--and the immigration rate will start high. When the island is empty, everything that shows up on it is a new species. But the immigration rate must inevitably fall down to zero when the number of species on the island equals the number in the source pool on the mainland.

• Because we're just counting species; we're not counting number of individuals arriving. I mean, if a hundred birds fly in and their species already exists on the island, that doesn't count for immigration, because that species is already there. So that's the way this axis is constructed.

• The number of species on the island is going to be affecting the extinction rate, probably in two ways. The simplest is the more species there are, the greater the chance that one of them will go extinct, just at random. So that curve's going to go up, just because there are more species on the island.

• However, if there are interactions among the species on the island, such that predation, disease, whatever is going to drive one to extinction, you can see that it might bend upward. So it's not just going to be linear, it's going to go up; so that's how they argued the curves.

• And they said where the curves intersect, the number coming in will equal the number going out, and that's the number we should expect to find on the island.?

What's going to affect the rates of immigration and extinction??

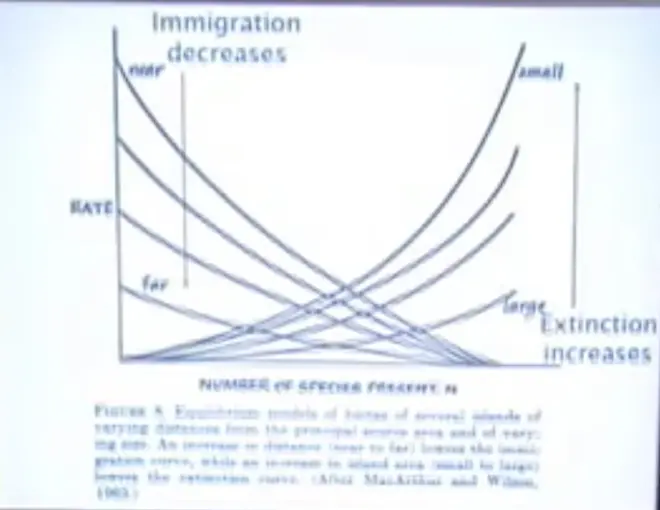
• Immigration will decrease?from islands that are near the mainland out to islands?that are far from the mainland. So you could construct a series of curves; so this would be the curve for an island close to the mainland and this would be the curve for an island far from the mainland. And that's just because it's harder to get out there.

• On the other hand,?extinction rates will increase as you go from large islands down to small islands, basically because there's more space on the big island, more different niches, more places, habitats, where organisms can live; more different kinds of things could survive there. But also as you get onto a small island, the intensity of the biotic interactions are going to get bigger, and it's going to be harder to get away from a predator, or harder to get away from a parasite. And so you could imagine that as you shrank an island, extinction rates would go up.

• We could have an equilibrium over here on an island which is small and far from the coast, or that we could have it here if it was a small island close to the continent. And similarly if we have a large island which is far away from the continent, we might be down here, and a large island close to the continent would be here.

So why is this important? Well for a long time, from the mid-1960s up to about 1985, 1990, this was the only game in town, and there weren't alternative ways of thinking about these processes, and it played a big role in the design of natural parks and nature reserves.?

• Essentially it said, because of the area, the effect of area on biodiversity, it's better to have a big park than a small one, and because of the effect of immigration rate on biodiversity, it said it's better to provide corridors to connect landscape fragments, if you possibly can, so that things can immigrate and move back and forth. So it was used a lot.

• However, when we summarize it, you'll see it's an equilibrium between colonization and extinction; it assumes there's a source population, that's where all the species are. There's no speciation occurring on islands. There are just two effects that you're worried about: how big the island is and how far away it is from the mainland. Extinction is driven by area, and colonization is driven by distance.

• If you're on a small island, a long way from the mainland, you'll have low species diversity, and a big island close to the mainland will have high species diversity.?

Now, as you'll see in a minute, I am now going to blast this theory out of the water. I'm going to take it apart and show that it makes a whole series of assumptions and claims that are demonstrably not true.?

• So the theory is dealing only with the number of species, not with the number of individuals; there's no population dynamics. If you've got ten of them on the island, or a thousand of them on the island, you count them the same way. That seems to be?silly, because the probably of extinction should be related to the number that are there.

• All the species are considered together, and there's just kind of one general immigration rate and one general extinction rate.?But the probabilities of immigration and extinction are different.?It's going to be different for birds and ants and mosses and paramecia and elephants.?So they must some ways differ systematically.

• The island biogeographic theory, kind of like the Hardy-Weinberg theory, it's an equilibrium theory.?It doesn't allow for history. But if we just look at what's happened in the Western Pacific in the last 10,000 years, as the Polynesians came out and colonized those islands, we know that 25% of the birds that were on them have already gone extinct.?It's a very misleading count because 25% extinction has already happened on those islands. This effect has not been compensated for in that dataset.

• The theory doesn't allow for speciation and adaptive radiation. And you take one pair of cardueline finches from Central America and fly them out to Hawaii, fifteen or twenty million years ago, and voilà, this is what they produce,?bunches of species. So that's going on, and that's not in the theory.

• It assumes that the probability of being able to immigrate doesn't depend on how many species are already there. But the presence of some species is probably a prerequisite for that of others, and the presence of some may keep others from coming in. So those are probably effects to worry about. It's actually empirically difficult to decide when an immigration has occurred.

• So you're sitting here, out in the Thimble Islands?off Branford, Connecticut, and it's spring, and a Bay-breasted Warbler comes flying through. Do you count it? Well it's going to just stop off, eat a few insects, and fly on to Canada. It's just passing through. Is that immigration? Probably not. So you have to actually find the species that breed on the island; that's not so easy.

• We assume the system's at equilibrium. But how can we recognize an equilibrium when we've got one? There's not a clear prediction on how fast this turnover would occur. Or do we have to wait ten generation, a hundred generations, a thousand generations? And hey, what about the problem that generation time is fast for little things and slow for big things? That makes that issue pretty complicated.

• If it is at equilibrium, the assumption is that every time a new species comes in, one that was already there goes extinct. Well that seems to be a little unrealistic. That's a very tight coupling of immigration and extinction, and the real relationship's weaker.?

So the major assumption of the theory, which is that there's a turnover of species that produces an equilibrium between immigration and extinction is correct. That's been tested experimentally on small islands. But the observed turnover is often of casual species, not the ones that breed with established populations, and there's nothing in the theory to tell us what proportion should be in each category. So the theory is a failure; the theory is a failure if the goal is to be right.

That's the island biogeography view of the universe. Now I want to do metapopulations, because this is the other major alternative way of looking at spatial dynamics of species and populations. So a metapopulation is a set of local populations that are linked by movement. And, as with island biogeography, the dynamics of metapopulations are driven by extinction and re- colonization, or immigration. So I'm going to be talking a bit about?frogs, butterflies, thyme plants, pathogen populations living in us, things like that.?

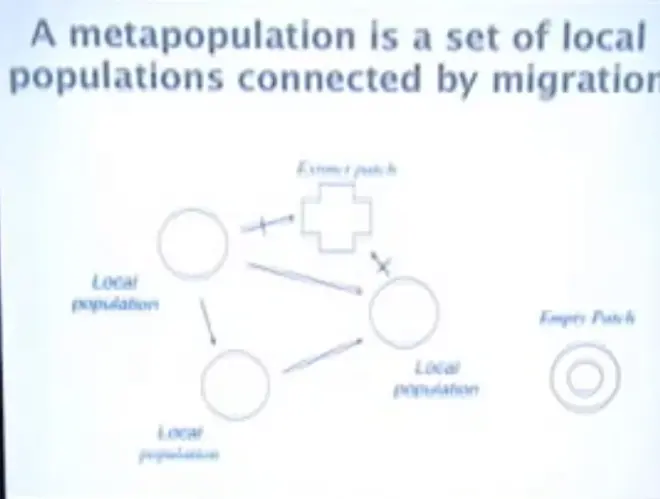
• So here's the basic conceptual framework. Here we have a local population and it's got reproduction going on in it. It's producing an excess of organisms, and they're moving out into the environment, because it's getting crowded locally and they want to find a place to live. They go out and they can find an empty patch to colonize.

• And sometimes, for one reason or another, their population will go extinct in a local patch. So if you just take a big sample of patches across the landscape, each of which is a population, you will find some of them with thriving biology going on, and some of them are empty; and they can be empty because they went extinct or they can be empty because they were never colonized. And all of them have the conditions that the organisms need to survive in.?

So if you build a simple metapopulation model, you can pull out some pretty important, straightforward messages.?

• Even if every single local population is likely to go extinct, the metapopulation can survive in a balance between extinction and colonization. So basically when they realize they are?about to go extinct locally;?they'd?better get up and fly out, and go find a new place, and just keep hopping. And if a population manages to do that, it can keep itself going, even though it leaves behind a long trail of consistent extinctions.

• The landscape is important in this, and of course that's very attractive because it gets people into landscape ecology; it gets them into photographs taken from space and?geographic information systems.?So the landscape features that are going to effect extinction and colonization then are going to be things that are very important for regional persistence. If we're dealing with say Daphnia living in ponds in Connecticut, and in any particular pond in Connecticut Daphnia is likely to go extinct, but we look across the whole state and we see that there are 100,000 ponds, Daphnia actually ends up probably doing just fine in Connecticut.

• Another message is that there is a ratio between colonization and extinction, above which a metapopulation can exist. So if you're concerned with the question, is there an analytically determinable threshold for our daphnia population in the State of Connecticut that will tell us how much they have to move around in order to stay here in the long run? The model will give us that. That's the threshold ratio between colonization and extinction.

• You can interpret the threshold?in terms of the proportion of patches that are occupied and average patch size. If you're concerned with population viability analysis, if you're concerned with conservation and with the threats to biodiversity, this is something that you can actually go out to measure, and then construct an argument with.

So here are a few insights.?

• It's perfectly normal to have some local empty patches. You need to?look?at the region and?the landscape, rather than the local population.?In Switzerland they were very worried about their carabid beetles.?And locally people would get very desperate about their local pond not having any salamanders in it anymore,?whereas if they would back up and they would look at say a chunk of landscape that was 100 kilometers on a side, they could relax, because local things are often going extinct but then being re-colonized. And you actually need to be able to back up and look at it, at a fairly large spatial scale, and a pretty long timescale, before you can discern the overall trend.

• There is something that's hard to measure and that's the migration rate.?It's just hard to see. After all, if I were a salamander, I would do it on a rainy night?at about two o'clock in the morning; and who's going to be out there tracking me around? So this is hard to measure.?

Is there evidence that in fact Nature is organized this way??

• We know that population size is significantly affected by migration, and we can see that in both the source effect and the sink effect. So you put a fence around a population, and if it's a source it will increase, and if it's a sink it will disappear.?And that can only happen if the source would normally be exporting migrants, immigrants that are going out, and the sink would only happen, it would only disappear, when the fence is put around it, if it had been previously maintained by stuff coming in from sources. So that's experimentally demonstrable.

• We know that population density is affected by the area and the isolation of the patch. Big patches tend to have slightly higher densities, and distant patches tend to have lower densities.

• If it's really a metapopulation, then population density should be going up and down?out of synchrony. If it's really tightly linked, and there's a tremendous amount of immigration, then you could just treat the thing as just one big population. But if it's a metapopulation, and some things are doing okay and some things are going extinct, then doing okay means going up, and going extinct means going down, at the same time. So they would be asynchronous; and that is often observed.

• Is there a population turnover? Do local populations go extinct and then get re-colonized from a source? And that's been observed, at least in one case, for snails living in ponds in the U.K. And that's done by taking samples of the mud in the bottom of the ponds and going back over many years and looking for the presence of snails. And they disappear and they come back, and they disappear and they come back.

• If you're a good naturalist and you know where your beast likes to live, then one often sees that suitable habitat is present, but it's empty.?And both in a plant population and in a butterfly metapopulation, both of these metapopulations persist?despite lots of local extinction.

• The risk of extinction in a metapopulation does depend on patch size. If you're in a small one, it's much more likely that you'll go extinct than if you're in a large patch. So the evidence for that is pretty strong. And the colonization rate depends on patch isolation; and that's true for most species.

• The colonization rate, depending on patch isolation, however, would be very important for elephants, rhinoceroses, bears, stuff like that. Okay? So you can see that there's a gradation based on dispersal ability, body size; lots of biology. Small isolated patches are likely to be empty. Big connected patches are likely to be full. Lots of evidence for that; that's certainly a straightforward prediction of the model.

• And a fugitive competitor can exist in a metapopulation.?A fugitive competitor is one that if you just put them into a local equilibrium population, they will get beaten out by the other species that's there. But if they are better at dispersing, while the other one is better at competing, they can keep jumping out and getting ahead of that. And that's been well investigated with Daphnia in the Finish Archipelago. Furthermore, I'm going to show you a shot of a prey species that would go extinct in a local population, but it can co-exist with its predator in a metapopulation; and I'll show that for two mites living in greenhouses.?

Now, the point that is made here is that when you shift from the equilibrium local population perspective, up to the metapopulation perspective, the complexities of spatial distribution will allow a lot more things to co-exist with each other. And that's true both for competition theory and it's true for predation theory.?

• So here's the Finnish Archipelago. It is?continuing to emerge from the water, because there's glacial rebound. After the Pleistocene, glaciers melted. They had depressed the underlying continental crust, and it is now rebounding and rising up. So these islands continue to come out of the water. And they are a lovely kind of landscape, filled with all sorts of interesting biology. They have on them, for example, six-foot long water snakes, that are about that thick, and they have lots of birds and other things.

• And on them they have little pools of fresh water, surrounded by this part of the Baltic, which is not really seawater, it's kind of brackish. But the Daphnia can't really survive in the sea; the salinity is still too high for them. So they move around among pools, on these islands, in places like this; this little island here might have ten or twenty pools on it. And they are moving around, among these islands, probably having their ephippia, which are their resting stages, borne on the feet of shorebirds that are flying.

• And there are at least two species of Daphnia that live in the Finish Archipelago. One of them is a better competitor, one of them is a better disperser, and they co-exist because what one lacks in competition ability, it makes up in dispersal ability. So if you have that kind of a tradeoff, you can generate a persistent metapopulation in a system like that.

The other is mites in a greenhouse. And this is Carl Huffaker's experiment, and he had a brilliant idea for a model system in which you could investigate the impact of spatial structure on predator/prey interactions. He had a herbivorous mite that likes to eat oranges?and a?predatory mite that eats the herbivorous mite.?

• And what he did was he constructed a model ecosystem that consisted of a whole bunch of oranges, interspersed with billiard balls; well obviously the herbivorous mite can't eat a billiard ball, but it can eat an orange. And then he altered the migration rates of the species by putting grease down, in between the two. So he had a system that you could actually put in your kitchen cabinet, that had a complete spatial ecosystem in it, and he could play with the parameters.

• And what Huffaker discovered is that he could have persistence of a predator and prey with spatial structure where if they were confined to a single orange they would both go extinct; first the prey would go extinct and then the predator would start.?

Since 1985, there's been an explosion of interest in metapopulations. And there are really two reasons for that.?

• One of them is that it is obvious that the landscape really is fragmented, and so metapopulation theory has become an organizing concept in conservation biology, where people try to maintain biodiversity at a landscape scale.

• It's much easier to study and manipulate a metapopulation than it is to manipulate an archipelago. So there's been a lot more progress doing experiments, like the ones that I showed you that Carl Huffaker did. That was actually before the theory came up; he was sort of a prophet way ahead of his time.?

And there's an analogy to epidemiology, and it's quite compelling; and we know that epidemiology works. So I now want to show you the analogy with epidemiology. And this is a connection now between ecology and infectious disease. So the host is a local patch.?Pathogens have local populations within hosts. Now what would constitute extinction? Extinction would be either you kill your host, so you die with it, or the host develops an immune response. You can go extinct for either reason; and by the way, if you're a pathogen, either is equally bad.?The disease transmission rate is equivalent to the migration rate.?

• Measles in big cities are a huge metapopulation with a continual input of young hosts that don't have any immune defense: babies. If measles could get onto an island like that, or any other infectious disease that causes a sterilizing immune response, as measles does, then it'll sweep in a wave through that island, and everybody will become immune before enough babies can be born to maintain the disease. So repeated extinction occurs.

• And as we go from fairly large islands, down to smaller islands, we have more and more months with no cases, until you get to the Falkland Islands, and over that nineteen year period there wasn't a single case of measles in the Falkland Islands. They must've been pretty worried about a ship coming in that had somebody with measles on it, but there was no case during that nineteen years.

• So diseases will tend to go extinct on little islands, and host populations will then lose both their acquired and their inherited resistance. And if then after many years the disease is reintroduced, the epidemic can really be catastrophic. So I think you already know that on Hispaniola, between--that's the Dominican Republic and Haiti--between 1492 and the late-1500s, a population of about half a million indigenous Americans was reduced to 300, by measles and by other diseases.?

So this is the rescue effect in a metapopulation and it's the rescue effect in epidemiology. And that--basically the pathogens are being rescued by babies, and the babies are born susceptible; they do not yet have an acquired immune reaction, they haven't built up the population of cells that will target that particular pathogen. And so that rescues the disease before it goes extinct. And there are enough of these babies coming in, in a city, so that the colonization rate, the transmission rate, and the number of occupiable sites, is high enough to keep that population going.?

So the take-home point on this lecture?is that geography is very important in ecology, and there have been a number of pretty big attempts to create analytical systems to deal with it. A lot of the world is fragmented.

Movement by organisms, among fragments, creates a dynamic across the whole landscape. And local extinctions and re-colonizations may be an entirely normal thing, and you can't really see that until you look at a big enough chunk of space, in a long enough period of time, to establish a metapopulation dynamic.?

And then finally I'd like to emphasize that the spread of disease, epidemiology, can be viewed as a metapopulation dynamic, and can be viewed as a model system within which to test metapopulation assumptions.?